Learning Objectives

Problem 1 of 3

The use of any calculator is NOT allowed for this question.

(a) Solve the equation algebraically, giving exact values for x, where 0 ≤ x < 2π.

(b) Give the general solution for this equation. (Solve over the set of real numbers giving exact value solutions.)

Solution ---------------------------------------------------------------

(a) From the problem given, we know that 2cos² x + 3cos x + 1 = 0 is represented in the same form as ax² + bx + c = 0 where a > 1 and that the answers is where 0 ≤ x < 2π.

With this, in our problem a=2, b=3, and c=1 (the coefficients of the equation).

So with that, we can factor it by multiplying a with c, and use two numbers when multiplied together to get the new value, and also the value of b, when added together. Use the two numbers to break down the middle number.

This is how it would look:

2cos² x + 3cos x + 1 = 0

· Multiply 2 to 1. To get 2.

· Then get two numbers when multiplied together, to get 2, and when added together to get 3. This is 2 and 1.

· Then you'll have 2cos² x + 2cos x + 1cos x + 1 = 0.

· Which can be factored into (2cosx + 1) (cosx + 1) = 0.

Therefore cosx = -1/2 or cosx = -1.

So now according to the unit circle, when cosx = -1/2, the exact value is 2π/3, and 4π/3. Also when cosx = -1, the exact value is π.

These answers are for where 0 ≤ x < 2π, which is what we want.

So the solution to part (a) of this question is:

x = 2π/3

x = 4π/3

x = π

(b) The answers for part (a), are only for a specific domain where. But now we're trying to solve it over the set of real numbers. The answers are infinite. Look at the unit circle, and pick a point on it. Now, imagine it has spun around a full time going clockwise or counterclockwise, you're in the same position. This is why the answers are infinite. Another example is, a clock. When it is 12 o'clock, and 24 hours past, it will still be 12 o'clock, but really more time has past since the first.

So we use a general solution to answer it. Since 2π, is a full circle, and rotates around one time, we can just add multiples of it to our answer, because you'll end up in exactly the same spot. The variable to represent this will be 'k' just for example. But we can also spin around backwards or clockwise, this is why there are negatives. So in order for that to work 'k' has to be a member of intergers of whole numbers (..-2, -1, 0, 1, 2..etc). This is written by k ε I, meaning k has to be member of the intergers. The 'ε' means member.

The general solution for the equation 2cos² x + 3cos x + 1 = 0 is:

x = 2π/3 + 2kπ ; k ε I

x = 4π/3 + 2kπ ; k ε I

x = π + 2kπ ; k ε I

Problem 2 of 3

The use of any calculator is NOT allowed for this question.

A trigonometric graph has one maximum value at the point (π/4, 6) and the next minimum value at the point (3π/4, -2).

(a) Sketch the graph showing at least two periods.

(b) Find an equation for this graph in terms of the cosine function.

(c) Find an equation for this graph in terms of the sine function.

Solution

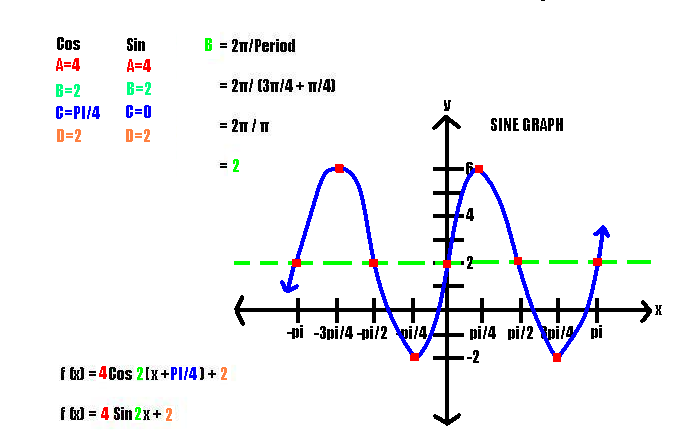
you always start off doing the great saying of DABC! the easiest way to approach these kinds of questions. in order to find D, you take the maximum y value and you add that to the minimun, then divide by 2, so that you can get the centre, which is the sinusodial axis. once you know this value, you can find out what A is by counting up or down from the sinusoldial axis. from there, we can find out the value of B by the formula B = 2pi / period. the period is the time it takes for one cycle. since we're given the max and the min value, we take both of the values, add them together and we have our period. so in this case, it was pi, so 2 pi / pi, the pi's reduce there for b = 2. if you know the basic sin graph, it starts at 0, then approaches 1, then 0, then -1, then 0 again. this graph starts at zero and goes down to -1, so that means the graph is negative if you use the point at 0 as the reference point. for C , that depends on which graph equation your oging to use, knowing which one you want, you pick a point on the graph which lies either on the sinusoldial axis, or the max or the min. with this done, you derive where the point would be if you had to make a equation for it. if the point is on the posistive side, in the equatoin you put a negative sign infront of the value in your equation. the two equations i derived, are just examples, if you find my writting not clear, refer to my diagram for clarification.

Problem 3 of 3

Consider the function y = sin(x) defined only on the interval .

(a) Sketch a clearly labeled graph of this function on its restricted domain.

(b) Sketch a clearly labeled graph of the inverse function y = sin^-1(x).

(c) State the domain of y = sin^-1(x).

(d) Evaluate sin^-1(-1/2).

Solution

note:descriptions are provided in diagrams/graphs

C) The domain of the equation is [-π/2, 0)U(0, π/2]

• We can read this interval as:

from and including, -π/2 to, not including, o

UNION

not including, 0, to including π/2

• We get -(π/2) and (π/2) because the inverse function is built from these "x-coordinates"
• We DO NOT include zero because zero is an asymptote of the this inverse function.